Dividing Complex Numbers

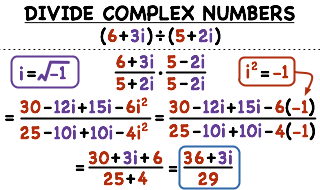
Dividing complex Numbers may look a little bit hard to you, but if you get the hang of it you will solve it in no time, Dividing is simply a Complex Number over a different Complex Number or an Imaginary Number or a Complex Number over a Real Number.As for the Complex Number or Imaginary Number, we will use the conjugate, which is reversing the sign of the Imaginary Part ONLY, and use the conjugate of them and multiply both the numerator and denominator with it, but if it is a a Real Number, then we deal with it as a normal simplify.

Lets look at the image up there, Let that equation be our example, we have (6+3i) ÷ (5+2i), first of all, write in the form of 6+3i / 5+2i, or as written in the image, then get the conjugate (reversing the sign of the Imaginary Number) of the Complex Number in the denominator's place, it will be 5-2i, after that multiply the original numerator and denominator with the conjugate, it will be like (6+3i / 5+2i) x (5-2i / 5-2i), or as written in the image, after doing the multiplication, combine alike terms together, then the result is 36+3i / 29 (keep it in fraction mode).

Notes: The Conjugte is the Reverse sign of the Imaginary Number.
Keep an eye to any Imaginary power units like i^(2) and replace them with their Value.